Section Modulus Calculator

Created by Luis Hoyos
Based on research by
Richard G. Budynas and J. Keith Nisbett “Roark's Formulas for Stress and Strain (Table A.1)“ McGraw-Hill Education (2020)See 2 more sources
Beer F, Johnston E, DeWolf J et al. “Mechanics of Materials, 7th Edition“ McGraw-Hill Education (2014)Hibbeler R “Mechanics of Materials 10th edition“ Pearson Educación (2017)
Last updated: Aug 24, 2022
Table of contents:

Calculating the section modulus is an interesting way to study the stress response of a beam to bending. With this tool, you'll be able to calculate the elastic and plastic section modulus of I-sections and many other common structural shapes.

If you don't know what is the section modulus, in the following article, we talk about it, the difference between the elastic and the plastic version, and the formulas for its calculation. Keep reading!

What is the elastic section modulus?

To know where the section modulus comes from, let's remember the widely known flexure formula used to determine the maximum stress in a beam subjected to bending:

σmax=McI=MI/c\sigma_{max} = \frac{Mc}{I} = \frac{M}{I/c}

where:

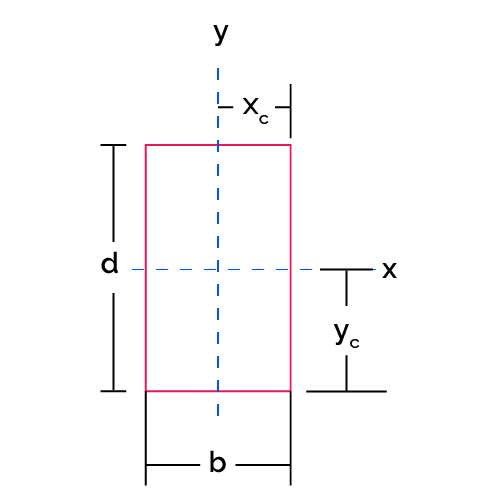
  • σmax\sigma_{max} — Maximum normal stress in the beam. This stress occurs at a distance cc from the neutral axis.
  • cc — Largest perpendicular distance from the neutral axis to a point in the section;
  • MM — Bending moment about the neutral axis; and
  • II — Area moment of inertia of the beam cross-section about the neutral axis.

Let's remember that this equation only applies if the beam meets the following conditions:

  • It is a prismatic beam (i.e., in the form of rectangular prism).
  • It is symmetrical about the axis perpendicular to the neutral axis.
  • Its material is homogenous and behaves according to Hooke's law.

For example, we can use the flexure formula for the following case:

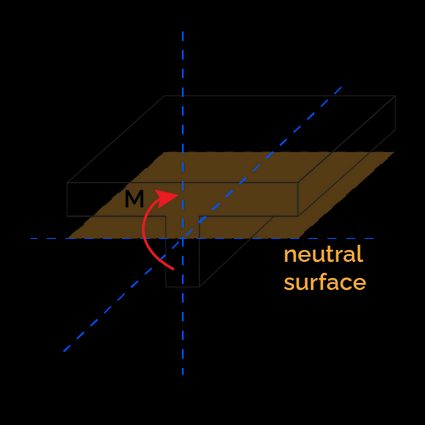
Beam under bending
Beam under bending.

Just like the area moment of inertia, the distance cc depends solely on the geometry of the section. We can combine II and cc and generate a new geometrical property that entirely accounts for the effects of the geometry on the normal stress. This property is known as the section modulus or elastic section modulus:

S=IcS =\frac{I}{c}

With this new property, we can now calculate the maximum normal stress due to bending by only knowing MM and SS:

σmax=MS\sigma_{max} = \frac{M}{S}

As well as for the moment of inertia, we can define the section modulus for the x and y directions. The axis to choose will depend on the direction of the momentum vector. For example, if the moment vector acts in the x-direction (the most common situation), we choose the section modulus in the x-direction.

Even so, if we face the situation of unsymmetric bending (bending in various directions or planes), we'll need to use methods that require the section modulus in both directions. In the last section of this article, you can look at a table with the different elastic and plastic section modulus formulas for different axes.

What is the plastic section modulus?

The plastic section modulus has a similar function to its elastic counterpart, but for situations in which we surpass the yield strength of the material and it no more exhibits a linear elastic behavior. The elastic section modulus makes it possible to calculate the plastic moment of the section:

Mp=ZσYM_p = Z\sigma_Y

where:

  • MpM_p — Plastic moment;
  • ZZ — Plastic section modulus; and
  • σY\sigma_Y — Yield strength of the beam material.

The plastic moment refers to the moment required to cause a plastic deformation across all the fibers of the beam section. As we know, for bending, the normal stress is higher at the points farthest from the neutral axis, and, therefore, it will reach the yield strength first at those points. Once the moment (M) equals the plastic moment (Mp), the yield strength will have reached the whole section.

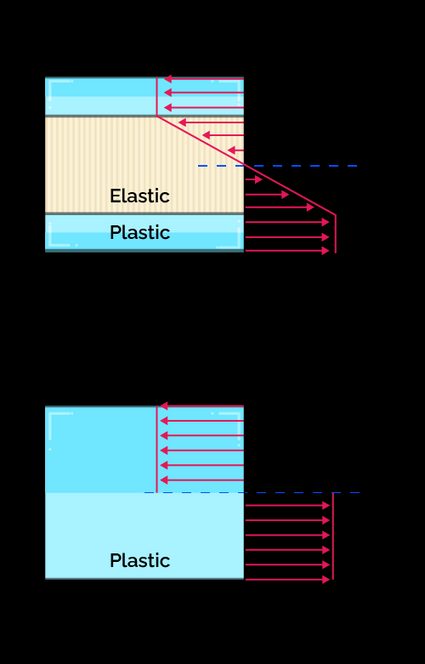
Plastic moment concept explanation.
Plastic moment concept explanation.

With the previous equation, we can easily predict the bending moment that will cause plastic deformation by only knowing the plastic section modulus and yield strength.

Now that we know the concepts let's look at the different formulas to calculate the section modulus.

Formulas to calculate the section modulus

In the following table, you can look a the elastic and plastic section modulus formulas, which are the ones this section modulus calculator uses. Scroll sideways to display the whole table.

Form

Elastic section modulus (S)

Plastic section modulus (Z)

Square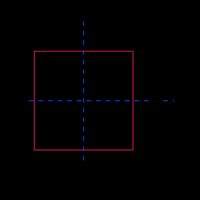

Image of a square section and its dimensions

yc=xc=a/2y_c = x_c = a/2

Zx=Zy=0.25a3Z_x = Z_y = 0.25a^3

Ix=Iy=a412I_x = I_y = \frac{a^4}{12}

Sx=Sy=Ixyc=a36S_x = S_y =\frac{I_x}{y_c} = \frac{a^3}{6}

Rectangle

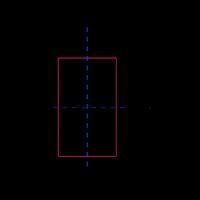Image of a rectangular section and its dimensions

yc=d/2y_c=d/2

Zx=0.25bd2Z_x = 0.25bd^2

xc=b/2x_c=b/2

Zy=0.25db2Z_y = 0.25db^2

Ix=bd312I_x = \frac{bd^3}{12}

Iy=db312I_y = \frac{db^3}{12}

Sx=Ixyc=bd26S_x = \frac{I_x}{y_c} = \frac{b d^2}{6}

Sy=Iyxc=db26S_y = \frac{I_y}{x_c} = \frac{d b^2}{6}

Hollow rectangle

Image of a hollow rectangle section and its dimensions

yc=d/2y_c=d/2

Zx=0.25(bd2−bidi2)Z_x = 0.25(bd^2-b_id_i^2)

xc=b/2x_c=b/2

Zy=0.25(db2−dibi2)Z_y = 0.25(db^2-d_ib_i^2)

Ix=bd3−bidi312I_x = \frac{bd^3-b_id_i^3}{12}

Iy=db3−dibi312I_y = \frac{db^3-d_ib_i^3}{12}

Sx=IxycS_x = \frac{I_x}{y_c}

Sy=IyxcS_y = \frac{I_y}{x_c}

Tee section

Image of a hollow tee section and its dimensions

yc=bt2+twd(2t+d)2(tb+twd)y_c=\frac{bt^2+t_wd(2t+d)}{2(tb+t_wd)}

If twd≥btt_wd \ge bt, then

xc=b/2x_c=b/2

Zx=d2tw4−b2t24tw−bt(d+t)2Z_x=\frac{d^2t_w}{4}-\frac{b^2t^2}{4t_w}-\frac{bt(d+t)}{2}

A=tb+twdA = tb + t_wd

If twd≤btt_wd \le bt, then

Ix=b(d+t)3−d3(b−tw)3         −A(d+t−yc)2I_x = \frac{b(d+t)^3-d^3(b-t_w)}{3} \\ \ \ \ \ \ \ \ \ \ - \footnotesize A(d+t-y_c)^2

Zx=t2b4−twd(t+d−twd/2b)2Z_x=\frac{t^2b}{4}-\frac{t_wd(t+d-t_wd/2b)}{2}

Iy=tb3+dtw312I_y = \frac{tb^3+dt_w^3}{12}

Zy=b2t+tw2d4Z_y= \frac{b^2t+t_w^2d}{4}

Sx=Ixd+t−ycS_x = \frac{I_x}{d+t-y_c}

Sy=IyxcS_y = \frac{I_y}{x_c}

Channel section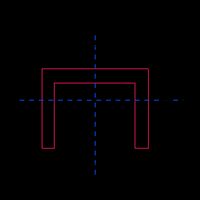

Image of a hollow rectangle section and its dimensions

yc=bt2+2twd(2t+d)2(tb+2twd)y_c=\frac{bt^2+2t_wd(2t+d)}{2(tb+2t_wd)}

If 2twd≥bt2t_wd \ge bt, then

xc=b/2x_c=b/2

Zx=d2tw2−b2t28tw−bt(d+t)2Z_x=\frac{d^2t_w}{2}-\frac{b^2t^2}{8t_w}-\frac{bt(d+t)}{2}

Ix=b(d+t)3−d3(b−2tw)3         −A(d+t−yc)2I_x = \frac{b(d+t)^3-d^3(b-2t_w)}{3} \\ \ \ \ \ \ \ \ \ \ -\footnotesize A(d+t-y_c)^2

If twd≤btt_wd \le bt, then

Iy=(d+t)b3−d(b−2tw)312I_y = \frac{(d+t)b^3-d(b-2t_w)^3}{12}

Zx=t2b4+twd(t+d−twdb)Z_x=\frac{t^2b}{4}+t_wd(t+d-\frac{t_wd}{b})

Sx=Ixd+t−ycS_x = \frac{I_x}{d+t-y_c}

Zy=b2t4+twd(b−tw)Z_y= \frac{b^2t}{4} + t_wd(b-t_w)

Sy=IyxcS_y = \frac{I_y}{x_c}

Wide-flange beam (equal flanges)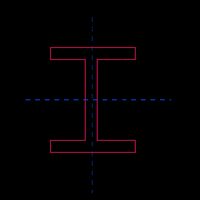

Image of a hollow rectangle section and its dimensions

yc=d/2+ty_c=d/2+t

Zx=twd24+bt(d+t)Z_x=\frac{t_wd^2}{4}+bt(d+t)

xc=b/2x_c=b/2

Zy=b2t2+tw2d4Z_y= \frac{b^2t}{2}+\frac{t_w^2d}{4}

Ix=b(d+2t)3−(b−tw)d312I_x = \frac{b(d+2t)^3-(b-t_w)d^3}{12}

Iy=b3t6+tw3d12I_y = \frac{b^3t}{6} + \frac{t_w^3d}{12}

Sx=IxycS_x = \frac{I_x}{y_c}

Sy=IyxcS_y = \frac{I_y}{x_c}

Angle

Image of a hollow rectangle section and its dimensions

yc=d2+bt+−t22(b+d−t)y_c=\frac{d^2+bt+-t^2}{2(b+d-t)}

If t≤A2bt \le \frac{A}{2b}, then

xc=b2+dt−t22(b+d−t)x_c=\frac{b^2+dt-t^2}{2(b+d-t)}

Zx=t(d−t)2−b2+2bd4Z_x=t\frac{(d-t)^2-b^2+2bd}{4}

A=t(b+d−t)A = t(b+d-t)

If t>A2bt > \frac{A}{2b}, then

Ix=bd3−(b−t)(d−t)33         −A(d−yc)2\footnotesize I_x = \frac{bd^3-(b-t)(d-t)^3}{3} \\ \ \ \ \ \ \ \ \ \ -A(d-y_c)^2

Zx=bt24+dt(d−t)2−t2(d−t)24bZ_x= \frac{bt^2}{4}+\frac{dt(d-t)}{2}-\frac{t^2(d-t)^2}{4b}

Iy=db3−(d−t)(b−t)33         −A(b−xc)2\footnotesize I_y = \frac{db^3-(d-t)(b-t)^3}{3} \\ \ \ \ \ \ \ \ \ \ -A(b-x_c)^2

Sx=Ixd−ycS_x = \frac{I_x}{d-y_c}

If t≤A2ht \le \frac{A}{2h}

Sy=Iyb−xcS_y = \frac{I_y}{b-x_c}

Zy=t(b−t)2−d2+2db4Z_y=t\frac{(b-t)^2-d^2+2db}{4}

If t>A2ht > \frac{A}{2h}

Zy=dt24+bt(b−t)2−t2(b−t)24dZ_y= \frac{dt^2}{4}+\frac{bt(b-t)}{2}-\frac{t^2(b-t)^2}{4d}

Circle

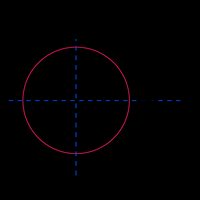Image of a circular section and its dimensions

yc=xc=Ry_c = x_c = R

Zx=Zy=1.333R3Z_x = Z_y = 1.333R^3

Ix=Iy=π4R4I_x = I_y = \frac{\pi}{4}R^4

Sx=Sy=Ixyc=π4R3S_x = S_y =\frac{I_x}{y_c} = \frac{\pi}{4}R^3

Hollow circle

Image of a hollow circular section and its dimensions

yc=xc=Ry_c = x_c = R

Zx=Zy=1.333(R3−Ri3)Z_x = Z_y = 1.333(R^3-R_i^3)

Ix=Iy=π4(R4−Ri4)I_x = I_y = \frac{\pi}{4}(R^4-R_i^4)

Sx=Sy=IxycS_x = S_y =\frac{I_x}{y_c}

Luis Hoyos
Inputs
Section
Rectangle
picture of a rectangle section
Base
in
Height
in
Rectangle
Sₓ
cu in
Sᵧ
cu in
Zₓ
cu in
Zᵧ
cu in
Formulas
In the "Section modulus formulas" text section, we present the formulas with which section modulus are calculated. If you think we're missing an important shape, let us know.
People also viewed…

Bend allowance

Use the bend allowance calculator to find the bend allowance and deduction of a metal sheet.
Bend Allowance Calculator

Hoop stress

Determine the stresses in thin walled shells (spheres and cylinders) using the hoop stress calculator.
Hoop Stress Calculator

Schwarzschild radius

Discover the fundamental of black hole physics with our Schwarzschild radius calculator.
Schwarzschild Radius Calculator
main background